Geometry pack: What can we learn from the Ancient Greeks about the order of Nature?
Use these six activities to explore the geometry of 3D shapes with students in KS2.
The six activities in this geometry pack have been developed to explore with students some of the discoveries the Ancient Greeks made about geometry and shape. They are linked to the Year 5 enquiry of learning What can we learn from the Ancient Greeks about the order of Nature? and can be used to support learning about 3D shapes in maths, to enrich an exploration of Ancient Greek civilisation or to teach geometry as a standalone activity. They could also be used to introduce students to the principle of Geometry.
In each activity, students focus on constructing a different 3D shape. Step-by-step text instructions are provided as a guide for teachers, with accompanying diagrams and lists of the resources students will need to complete each activity. There are also photocopiable templates to support the activities.
At the end of each activity, students could be encouraged to count how many faces, edges and vertices each of the shapes they have constructed has to consolidate their understanding of these concepts in maths.
To help students get the most out of these activities, we recommend the use of Jakar compasses, which can be purchased at a discounted rate on our website.
What’s in this geometry teaching pack?
The following six activities are included in this pack:
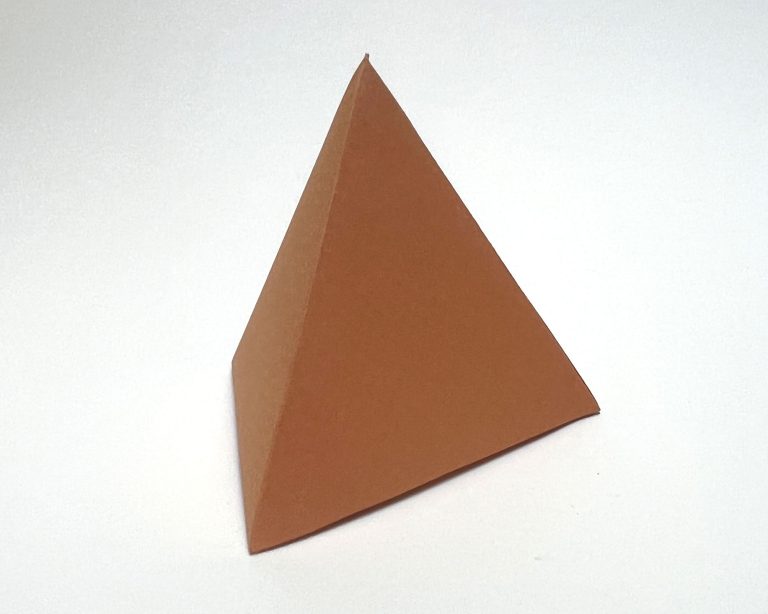
How do I build a tetrahedron?
The tetrahedron is one of the five Platonic solids. Platonic solids are special 3D shapes that share certain features: all the faces are identical regular polygons; exactly the same number of faces meet at each vertex; and every edge is the same length. In this activity, students use the geometry of circles to create a tetrahedron net.
How do I build a cube?
In this activity, students create a net for a cube – a regular polyhedron. It has six square faces, 12 edges and eight vertices. The cube is a symmetrical shape so no matter how you turn or flip it, it will always look the same. It has multiple lines of symmetry that divide it into equal parts.
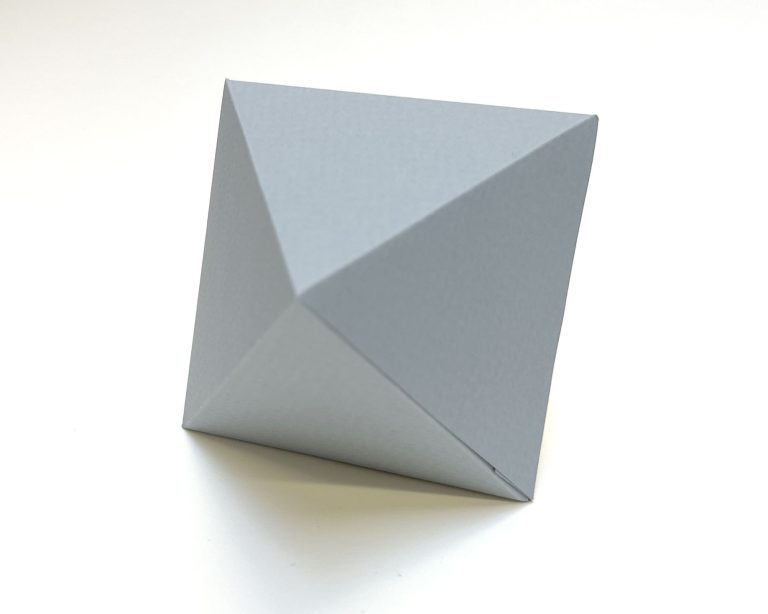
How do I build an octahedron?
Building on the skills developed in Activity 1, students use the geometry of circles to draw a net for an octahedron in this activity. This Platonic solid has eight identical triangular faces and looks a bit like two pyramids stuck together at their bases. It has 12 edges and six vertices.
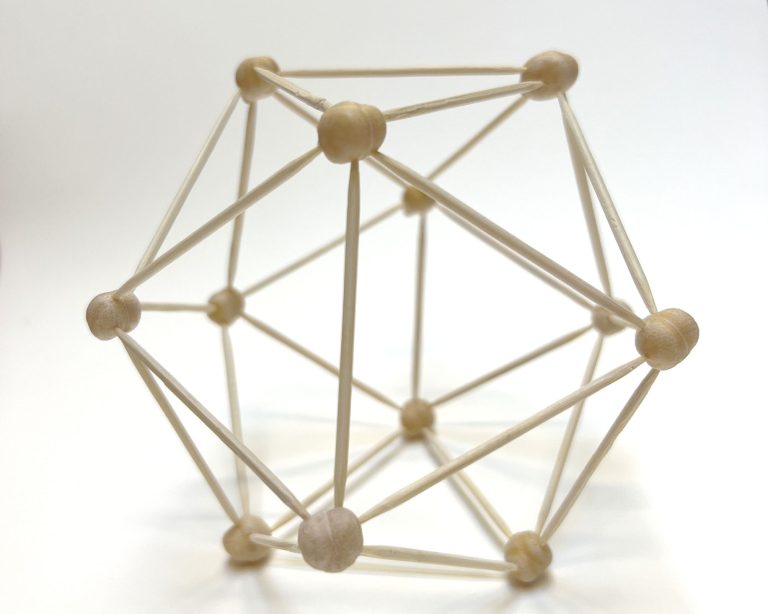
How do I build an icosahedron?
One of the Platonic solids, the icosahedron is an interesting geometric shape because it has a large number of faces – 20 in total, each of which is an equilateral triangle. This gives it a more spherical appearance compared with some other polyhedra. Students create their own icosahedron in this activity using toothpicks held together with chickpeas.
How do I build a dodecahedron?
The dodecahedron is another of the Platonic solids with 12 identical, regular pentagonal faces. In this activity, students work in pairs to create two nets that, when combined, form a dodecahedron. These nets are based on the geometry of pentagons and pentagrams.
How do I build an Archimedean solid?
An Archimedean solid is a special kind of 3D shape with faces that are different regular polygons – for example, triangles, squares or hexagons. As the sides of these shapes are all the same length, they fit together perfectly. The truncated tetrahedron is one of the Archimedean solids and in this activity, students construct a net for one based on the geometry of hexagons and triangles.
